Today we took a more detailed look at the diffusion equation to understand it's nature and behavior, in preparation for understanding its origin. Our starting point was to review last week's idea that the concentration profile evolves according to how "concave" the concentration profile is. As Caleb noticed, the concentration at any given point is decreasing when the concentration profile there is concave down, and vice versa.

Before we got to that though, we talked more about why we care about diffusion:

Understanding diffusion will help us understand fluid mechanics, because the diffusion of particles is very similar to the concept of the diffusion of momentum, which is a big part of fluid mechanics;
I showed an example from my workplace of mixing in a chemical reactor tank where I had prepared plots very similar to the ones we have been studying at Cogitania. Here's the animation we discussed that applied to real life research:

Uploaded by J. Alex Lee on 2017-05-01.

During our background discussion, we found another very interesting path thanks to Luka's idea that diffusion must be related to entropy, which is "the amount of disorder" in a system. We described a more useful way to think of entropy so that we can relate it to the thermodynamic reasoning for why diffusion should occur in the context of the laws of thermodynamics which state that processes should proceed in the direction of increasing entropy. In the photo, there's a gridded box that represents the space where diffusion is happening. If particles could only occupy one grid at a time, we asked how many different ways can we arrange 3 particles such that they are always within 1 space of each other (concentrated in one region of the box). We then asked how many different ways can we arrange them such that they are spaced farther apart.

One way to think about entropy is a way of comparing the number of microscopic states that could describe the situations we care about. In this case, there are more ways to arrange 3 particles far apart from each other (diffused; higher entropy) than ways to arrange them bunched together (concentrated; lower entropy). This helps us understand why the system prefers the diffused state rather than the concentrated state based on entropy arguments: systems tend to evolve toward higher entropy states, but importantly, doesn't give us an explanation of how fast the system should evolve from state to state, which is what we have been concerning ourselves with so far.

Actually, non-equilibrium thermodynamics can describe such rates, and this is yet another way to describe the same physics, bringing our tally up to three: (1) particle dynamics, as we have played with in our solar system simulator and which can help us simulate Brownian motion, (2) continuum dynamics, where the diffusion equation [main topic of the day] lives, and (3) Boltzmann dynamics, which is a typical name for the idea that Luka had. Although it is still relatively rare in applied fluid mechanics, there is at least one large commercial fluid simulation software by Exa that uses such methods.

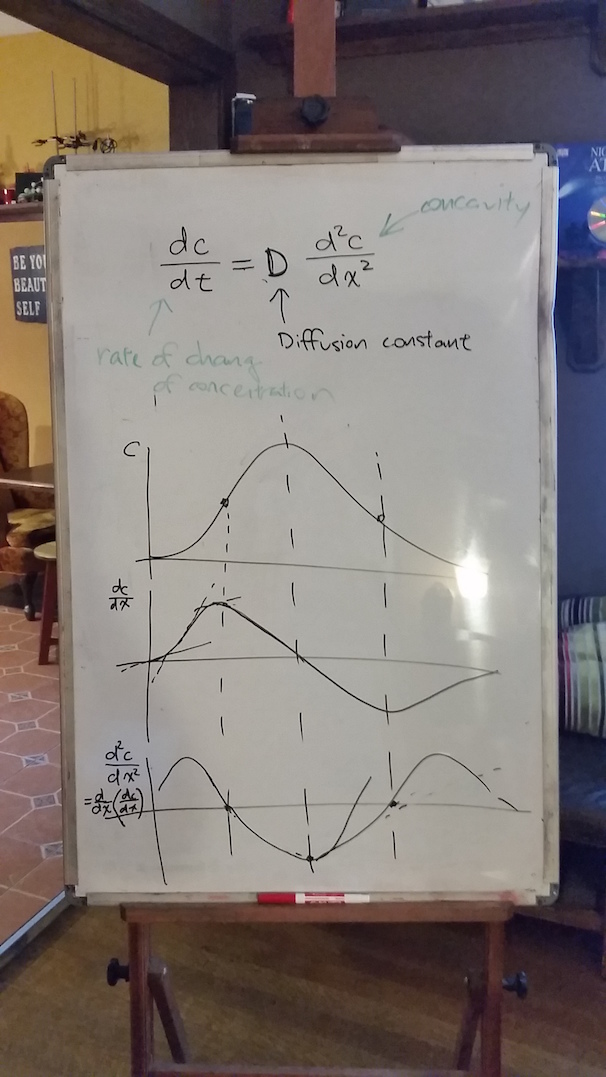
Now, back to the continuum diffusion equation. We worked on understanding how to describe "concavity" in quantitative terms. Of course, this meant back to calculus and derivatives. Specifically, we developed together the idea that concavity must be related to the second derivative of the concentration with respect to the position---this would at least give it the properties that we were describing in words: it is negative where the curve is concave down, positive where it is concave up, and there will be inflection points where it is neither concave up nor concave down

We drew the concentration profile, its first derivative, and second derivative on the white board as in the photo, but I promised more "correct" versions, so here they are:

Uploaded by J. Alex Lee on 2017-05-01.

Wherever the second derivative is positive, we should be able to see that the concentration itself is increasing (and vice versa). So as the top of the white board shows, we have related the rate that concentration changes (at some location) to the second derivative of the concentration with respect to its location there. This is exactly the diffusion equation, which we have now described somewhat more formally based on Caleb's original idea.

Why should the rate of concentration change be related to the 2nd derivative at all? What is the physics behind this relationship? Can you find the answer on Wikipedia?

The next question is: why should the rate of concentration change be related to the 2nd derivative at all? What is the physics behind this relationship? This question is often neglected (see if you can find the answer to this question on the Wikipedia), and perhaps for the good reason that its presentation typically requires a highly developed abstract intuition about the calculus of vectors. But does it really? We will try to build up this intuition next time we meet.

Thanks!
— Alex

April 30 applied physics review